Neil G4DBN
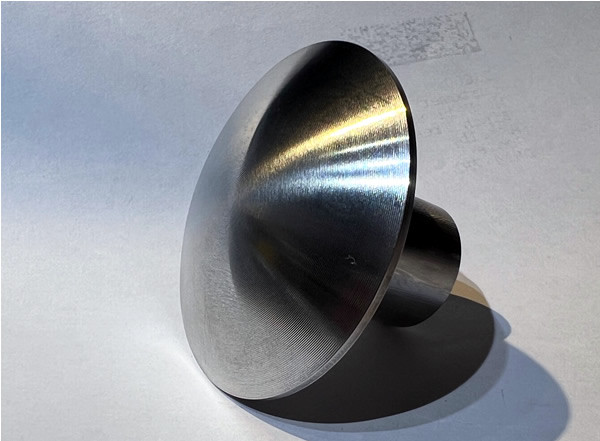
g4dbn@mastodon.radioWhen I'm designing a Cassegrain antenna for mmWave radio, the main dish is a parabola, but the deep ones from Edmund Optics are very hard to illuminate because they have a focal length to diameter ratio of 0.25, which is WAAAAAY less than most dishes. Solution is to put a hyperboloidal subreflector with its rear focus coincident with the prime focus of the parabola, and the front focus of the hyp is where you put the feedhorn. I made the rear so it can take a very fine adjustment thread
Neil G4DBN
g4dbn@mastodon.radioWhen I create the hyperbolic curve, it is described the usual mathematical way, with just the values of a and b from the standard equation of a vertical hyperbola. Problem is that Fusion CAD describes conic curves in terms of the rho value and the height and width of the tangent intercepts. I guessed there would be a conversion function. HAHAHAHAHA nope. So I sat and did a load of calculus and trig and algebra, resorting to Wolfram Alpha at one point. All sorted, and here is the first reflector
Neil G4DBN
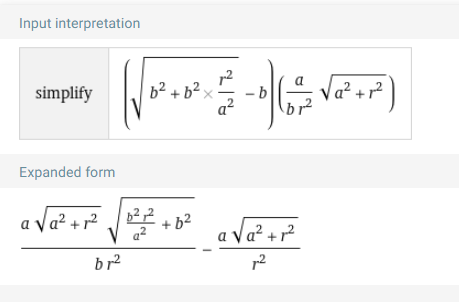
g4dbn@mastodon.radioAfter working everything out and doing the usual simple chain rule differentiation, I ended up with a horrible mess. Excellent, I thought. Use the simplify operations in Wolfram to distil it down to a nice, sensible expression with a few terms. After all, it's just a hyperbola. Two real positive numbers define it completely. Well played, Wolfram Alpha. Well played.
Neil G4DBN
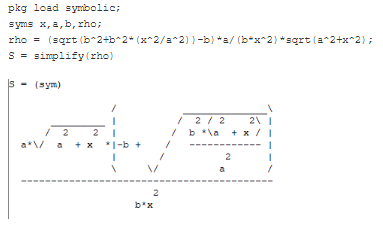
g4dbn@mastodon.radioSo THEN, I tried it in MATLAB, well, Gnu Octave. Really nice terminal style output. Elegant and simple. Also complete fail, but I can forgive it because it was almost apologetic.
Neil G4DBN
g4dbn@mastodon.radio@jmorris Working on a full range of items for sale, SMA, 2.92, N and TNC to waveguide transitions, waveguide to waveguide tapered transitions, Cassegrain and Gregorian subreflectors for multiple dishes and frequencies, directional couplers, waveguide and coaxial filters, Edmund Optics dish mounts and focus slides and hopefully some high precision machined dishes for mmWave, parabolic but also axially-displaced ellipse types, lots of feedhorns and lenses. No time to sleep or make Youtube vids!